In geometry, the stereographic projection, usually denoted by  , is a particular mapping (function) that projects a sphere onto a plane. The projection is defined on the entire sphere, except at one point – the projection point. Where it is defined, the mapping is smooth and bijective. It is conformal, meaning that it preserves angles. It is neither isometric nor area-preserving: that is, it preserves neither distances nor the areas of figures.
, is a particular mapping (function) that projects a sphere onto a plane. The projection is defined on the entire sphere, except at one point – the projection point. Where it is defined, the mapping is smooth and bijective. It is conformal, meaning that it preserves angles. It is neither isometric nor area-preserving: that is, it preserves neither distances nor the areas of figures.
Intuitively, then, the stereographic projection is a way of picturing the sphere as the plane, with some inevitable compromises. Because the sphere and the plane appear in many areas of mathematics and its applications, so does the stereographic projection; it finds use in diverse fields including complex analysis, cartography, geology, and photography. In practice, the projection is carried out by computer or by hand using a special kind of graph paper called a stereonet or Wulff net.

In Cartesian coordinates  on the sphere
on the sphere 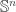 and
and 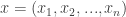 on the plane, the projection
on the plane, the projection 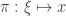 and its inverse
and its inverse  are given by the formulas
are given by the formulas

and
 .
.
Let us show you an example making use of the projection. We assume 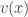 verifies the following PDE
verifies the following PDE
 .
.
Then the transformed function  , to be exact
, to be exact  , given by
, given by

satisfies the following PDE
 .
.
where 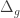 denotes the Laplace-Beltrami operator with respect to the standard metric
denotes the Laplace-Beltrami operator with respect to the standard metric  on
on 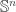 .
.
Similarly, if function  verifying the PDE
verifying the PDE

then a new function 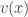 given by
given by

will satisfy the following PDE
 .
.
More general, PDE

becomes
 .
.
In conclusion, using the stereographic projection we can transfer some geometric problems on sphere 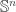 to ones in the whole space
to ones in the whole space  .
.
the stereographic projection performed with
as the north pole to the equatorial plane of
. Clearly when
is the north pole
, i.e.
, then
is the usual stereographic projection.
, the image of
is
and
are easy, for interested reader, I refer to an answer in
![]() . Let us now define the usual conformal transformation
. Let us now define the usual conformal transformation given by
