Ngô Quốc Anh

June 11, 2011

The Entropy-Logarithmic energy inequality

Filed under: PDEs, Riemannian geometry — Tags: — Ngô Quốc Anh @ 17:39

Recently, I have read a result by J. Demange published in J. Funct. Anal. in 2008 [here]. In that paper,  as a simple consequence, the author proves the following interesting inequality

Theorem. Let n\geqslant 2 be an integer and M be a compact connected n-dimensional Riemannian manifold with Ricci curvature bounded below by a positive constant \rho. The following inequality holds for d \in \mathbb R, d>n, d \geqslant 3, \alpha=1/2-1/d, and f , a smooth function mapping M onto \mathbb R_+

\displaystyle \left(\int_M f\right)^{2\alpha}-\int_M f^{2\alpha} \leqslant \frac{1}{K(n,d)}\int_M |\nabla f^\alpha|^2

and

\displaystyle \left(\int_M f\right)^{2\alpha}-\int_M f^{2\alpha} \leqslant K_1\log\left(1+K_2\int_M |\nabla f^\alpha|^2\right)

where

\displaystyle K(n,d)=\frac{\rho (d-2)}{4(1-\frac{1}{n})}, \quad L(n,d)=\frac{d-n}{n+2}\left( 4-9\frac{d-n}{d(n+2)}\right),

and

\displaystyle K_2=\frac{L(n,d)d}{4(d-2)K(n,d)(\int_M f)^{2\alpha}},\quad \frac{1}{K_1}=K_2K(n,d).

(more…)

April 22, 2011

On Costa-Hardy-Rellich inequalities

Filed under: Giải Tích 2, Giải Tích 6 (MA5205) — Tags: , , , — Ngô Quốc Anh @ 15:44

This note is to concern a recent result by David G. Costa [here]. Here the statement

Theorem 1.1. For all a,b\in \mathbb R and u \in C^\infty_0(\mathbb R^N\backslash\{0\}) one has

\displaystyle\left| {\frac{{N - 2 - \gamma }}{2}\int_{\mathbb R^N} {\frac{{|\nabla u{|^2}}}{{|x{|^\gamma }}}dx} + \gamma \int_{\mathbb R^N} {\frac{{{{(x \cdot \nabla u)}^2}}}{{|x{|^{\gamma + 2}}}}dx} } \right| \leqslant {\left( {\int_{\mathbb R^N} {\frac{{|\Delta u{|^2}}}{{|x{|^{2b}}}}dx} } \right)^{\frac{1}{2}}}{\left( {\int_{\mathbb R^N} {\frac{{|\nabla u{|^2}}}{{|x{|^{2a}}}}dx} } \right)^{\frac{1}{2}}}

where \gamma=a+b+1. In addition, if \gamma \leqslant N-2, then

\displaystyle\widehat C\int_{\mathbb R^N} {\frac{{{{(x \cdot \nabla u)}^2}}}{{|x{|^{\gamma + 2}}}}dx} \leqslant {\left( {\int_{\mathbb R^N} {\frac{{|\Delta u{|^2}}}{{|x{|^{2b}}}}dx} } \right)^{\frac{1}{2}}}{\left( {\int_{\mathbb R^N} {\frac{{|\nabla u{|^2}}}{{|x{|^{2a}}}}dx} } \right)^{\frac{1}{2}}}

where the constant \widehat C=|\frac{N+a+b-1}{2}| is sharp.

Here’s the proof.

(more…)

January 6, 2011

The Alexandrov-Bol inequality

Filed under: Giải Tích 6 (MA5205) — Tags: , — Ngô Quốc Anh @ 19:55

In the literature, there is an inequality called the Alexandrov-Bol inequality which is frequently used in partial differential equations. Here we just recall its statement without any proof.

Theorem. Let \Omega be a good domain in \mathbb R^2. Assume p \in C^2(\Omega)\cap C^0(\overline \Omega) be a positive function satisfying the elliptic inequality

\displaystyle -\Delta \log p \leqslant p

in \Omega. Then it holds

\displaystyle l^2(\partial\Omega) \geqslant \frac{1}{2} \big(8\pi-m(\Omega)\big)m(\Omega)

where

\displaystyle l(\partial\Omega)=\int_{\partial\Omega}\sqrt{p}ds

and

\displaystyle m(\Omega)=\int_\Omega pdx.

An analytic proof was given by C. Bandle aroud 1975 when she assumed p to be real analytic. The above version was due to Suzuki in an elegant paper published in the Ann. Inst. H. Poincare in 1992 [here]. The proof is mainly depended on the isoperimetric inequality for the flat Riemannian surfaces. We refer the reader to the paper by Suzuki for the proof.

December 15, 2010

The Cheeger isoperimetric constant

Filed under: Uncategorized — Tags: — Ngô Quốc Anh @ 19:30

In Riemannian geometry, the Cheeger isoperimetric constant of a compact Riemannian manifold M is a positive real number h(M) defined in terms of the minimal area of a hypersurface that divides M into two disjoint pieces of equal volume. In 1970, Jeff Cheeger proved an inequality that related the first nontrivial eigenvalue of the Laplace-Beltrami operator on M to h(M). This proved to be a very influential idea in Riemannian geometry and global analysis and inspired an analogous theory for graphs.

The Cheeger constant. Let M be an n-dimensional closed Riemannian manifold. Let V(A) denote the volume of an n-dimensional submanifold A and S(E) denotes the n-1-dimensional volume of an submanifold E (commonly called “area” in this context).

Definition. The Cheeger isoperimetric constant of M is defined as

\displaystyle h(M)=\inf_E \frac{S(E)}{\min(V(A), V(B))},

where the infimum is taken over all smooth n-1-dimensional submanifolds E of M which divide it into two disjoint submanifolds with boundary A and B. Isoperimetric constant may be defined more generally for noncompact Riemannian manifolds of finite volume.

It is well-known that the Cheeger constant h(M) can be characterized by the following

\displaystyle h(M): = \mathop {\inf }\limits_{f \in C_0^\infty (M)\backslash \{ 0\} } \frac{{\int_M {\left| {\nabla f} \right|d\mu } }}{{\int_M {\left| f \right|d\mu } }}.

The Cheeger inequality. The Cheeger constant h(M) and \lambda_1(M), the smallest positive eigenvalue of the Laplacian on M, are related by the following fundamental inequality proved by Jeff Cheeger

\displaystyle \lambda_1(M)\geq \frac{h^2(M)}{4}.

This inequality is optimal in the following sense: for any h>0, natural number k and \varepsilon>0, there exists a two-dimensional Riemannian manifold M with the isoperimetric constant h(M) = h and such that the kth eigenvalue of the Laplacian is within \varepsilon from the Cheeger bound (Buser, 1978).

Following is the proof of the Cheeger inequality. I found this proof from a book due to Alexander Grigoryan (problem 10.8).

Proof. Replacing f by f^2 we obtain

\displaystyle h(M) \leqslant \frac{{\int_M {\left| {\nabla ({f^2})} \right|d\mu } }}{{\int_M {{f^2}d\mu } }} = 2\frac{{\int_M {\left| f \right|\left| {\nabla f} \right|d\mu } }}{{\int_M {{f^2}d\mu } }} \leqslant 2\frac{{{{\left\| f \right\|}_{{L^2}}}{{\left\| {\nabla f} \right\|}_{{L^2}}}}}{{\left\| f \right\|_{{L^2}}^2}} = 2\frac{{{{\left\| {\nabla f} \right\|}_{{L^2}}}}}{{{{\left\| f \right\|}_{{L^2}}}}}.

Taking \inf in f, we obtain the desired inequality.

The Buser inequality. Peter Buser proved an upper bound for \lambda_1(M) in terms of the isoperimetric constant h(M). Let M be an n-dimensional closed Riemannian manifold whose Ricci curvature is bounded below by -(n-1)a^2, where a \geqslant 0. Then

\displaystyle\lambda_1(M)\leq 2a(n-1)h(M) + 10h^2(M).

For a proof of the Buser inequality, we refer the reader to a paper due to Ledoux published in Proc. Amer. Math. Soc. [here].

Source: WikiPedia

October 11, 2010

The Agmon type inequality in 1D

Filed under: Uncategorized — Tags: , , — Ngô Quốc Anh @ 18:14

In this note, we prove a very interesting inequality known as the Agmon type inequality in space dimension 1.

\displaystyle |g(x){|^2} \leqslant {\left( {\int_\mathbb{R} {|g(\xi ){|^2}d\xi } } \right)^{\frac{1}{2}}}{\left( {\int_\mathbb{R} {|g'(\xi ){|^2}d\xi } } \right)^{\frac{1}{2}}},\quad \forall x \in \mathbb{R}

for any smooth function g with compact support in \mathbb R.

The proof is standard and classical. The trick is to use the integral representation for functions that we have already discussed when we talk about the Poincare inequality.

Proof. Since g has compact support, there exists some L>0 sufficiently large such that g vanishes outside of (-L,L). Then we can write

\displaystyle g{(x)^2} = 2\int_{ - L}^x {g(\xi )g'(\xi )d\xi } \leqslant 2\int_{ - L}^x {|g(\xi )||g'(\xi )|d\xi }

and

\displaystyle g{(x)^2} = -2\int_x^{ L}{g(\xi )g'(\xi )d\xi } \leqslant 2\int_x^{L}{|g(\xi )||g'(\xi )|d\xi }.

Adding both inequalities we obtain

\displaystyle g{(x)^2} \leqslant \int_{ - L}^L {|g(\xi )||g'(\xi )|d\xi } = \int_\mathbb{R} {|g(\xi )||g'(\xi )|d\xi }

Then using the Cauchy-Schwarz inequality we find the desired inequality.

I will show some application of this equality, precisely, I will derive a proof of the Ladyzhenskaya inequalities

\displaystyle \int_{{\mathbb{R}^2}} {|u(x){|^4}dx} \leqslant \left( {\int_{{\mathbb{R}^2}} {|u(x){|^2}dx} } \right)\left( {\int_{{\mathbb{R}^2}} {|\nabla u(x){|^2}dx} } \right)

and

\displaystyle \int_{{\mathbb{R}^3}} {|u(x){|^4}dx} \leqslant {\left( {\int_{{\mathbb{R}^3}} {|u(x){|^2}dx} } \right)^{\frac{1}{2}}}{\left( {\int_{{\mathbb{R}^3}} {|\nabla u(x){|^2}dx} } \right)^{\frac{3}{2}}}

which plays an important role in the theory of Navier-Stokes equations.

May 9, 2010

The lowest eigenvalue of the Laplacian for the intersection of two domains

Filed under: PDEs — Tags: , , — Ngô Quốc Anh @ 5:59

Let us begin with a discussion of the geometric question. If A is an open set in \mathbb R^n (bounded or unbounded), let \lambda(A) denote the lowest eigenvalue of -\Delta in A with Dirichlet boundary conditions. \lambda(A)=-\infty if A is empty. Intuitively, if \lambda(A) is small then A must be large in some sense. One well known result in this direction is the Faber-Krahn inequality which states that among all domains with a given volume |A|, the ball has the smallest \lambda. Thus,

\displaystyle\lambda(A) \geqslant \beta_n\frac{1}{|A|^\frac{2}{n}}

where \beta_n is the lowest eigenvalue of a ball of unit volume. This inequality clearly does not tell the whole story. If \lambda(A) is small then A must not only have a large volume, it must also be “fat” in some sense.

Let us place here a very beautiful result due to Lieb among other big contributions. This result was published in Invent. Math. during 1983. The proof relies upon the Rayleigh quotient and a very clever choice of a trial function for the variational characterization of \lambda(A\cap B_x). For the whole paper, we refer the reader to here.

Theorem. Let A and B be non-empty open sets in \mathbb R^n (n\geqslant 1), and \lambda(A) and \lambda(B) be the lowest eigenvalue of -\Delta with Dirichlet boundary conditions. Let B_x denote B translated by x\in \mathbb R^n. Let \varepsilon>0. Then there exists an x such that

\displaystyle\lambda(A \cap B_x)<\lambda(A)+\lambda(B) +\varepsilon.

If A and B are both bounded then there is an x such that

\displaystyle\lambda(A \cap B_x)<\lambda(A)+\lambda(B) .

Before proving the theorem, let us recall the so-called Rayleigh quotient. Precisely

(more…)

February 22, 2010

The Poincaré inequality: W^{1,p} vs. W_0^{1,p}

Filed under: Giải Tích 6 (MA5205), Giải tích 8 (MA5206), Nghiên Cứu Khoa Học, PDEs — Tags: — Ngô Quốc Anh @ 1:50

In mathematics, the Poincaré inequality is a result in the theory of Sobolev spaces, named after the French mathematician Henri Poincaré. The inequality allows one to obtain bounds on a function using bounds on its derivatives and the geometry of its domain of definition. Such bounds are of great importance in the modern, direct methods of the calculus of variations. A very closely related result is the Friedrichs’ inequality.

This topic will cover two versions of the Poincaré inequality, one is for W^{1,p}(\Omega) spaces and the other is for W_o^{1,p}(\Omega) spaces.

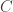The classical Poincaré inequality for W^{1,p}(\Omega) spaces. Assume that 1\leq p \leq \infty and that \Omega is a bounded open subset of the n–dimensional Euclidean space \mathbb R^n with a Lipschitz boundary (i.e., \Omega is an open, bounded Lipschitz domain). Then there exists a constant C, depending only on \Omega and p, such that for every function u in the Sobolev space W^{1,p}(\Omega),

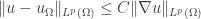\displaystyle \| u - u_{\Omega} \|_{L^{p} (\Omega)} \leq C \| \nabla u \|_{L^{p} (\Omega)},

where

\displaystyle u_{\Omega} = \frac{1}{|\Omega|} \int_{\Omega} u(y) \, \mathrm{d} y

is the average value of u over \Omega, with |\Omega| standing for the Lebesgue measure of the domain \Omega.

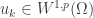Proof. We argue by contradiction. Were the stated estimate false, there would exist for each integer k = 1,... a function u_k \in W^{1,p}(\Omega) satisfying

\displaystyle \| u_k - (u_k)_{\Omega} \|_{L^{p} (\Omega)} \geq k \| \nabla u_k \|_{L^{p} (\Omega)}.

We renormalize by defining

\displaystyle {v_k} = \frac{{{u_k} - {{({u_k})}_\Omega }}}{{{{\left\| {{u_k} - {{({u_k})}_\Omega }} \right\|}_{{L^p}(\Omega )}}}}, \quad k \geqslant 1.

Then

\displaystyle {({v_k})_\Omega } = 0, \quad {\left\| {{v_k}} \right\|_{{L^p}(\Omega )}} = 1

and therefore

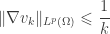\displaystyle\| \nabla v_k \|_{L^{p} (\Omega)} \leqslant \frac{1}{k}.

In particular the functions \{v_k\}_{k\geq 1} are bounded in W^{1,p}(\Omega).

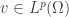By mean of the Rellich-Kondrachov Theorem, there exists a subsequence {\{ {v_{{k_j}}}\} _{j \geqslant 1}} \subset {\{ {v_k}\} _{k \geqslant 1}} and a function v \in L^p(\Omega) such that

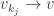\displaystyle v_{k_j} \to v in L^p(\Omega).

Passing to a limit, one easily gets

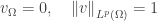\displaystyle v_\Omega = 0, \quad {\left\| {{v}} \right\|_{{L^p}(\Omega )}} = 1.

On the other hand, for each i=\overline{1,n} and \varphi \in C_0^\infty(\Omega),

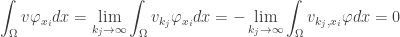\displaystyle\int_\Omega {v{\varphi _{{x_i}}}dx} = \mathop {\lim }\limits_{{k_j} \to \infty } \int_\Omega {{v_{{k_j}}}{\varphi _{{x_i}}}dx} = - \mathop {\lim }\limits_{{k_j} \to \infty } \int_\Omega {{v_{{k_j},{x_i}}}\varphi dx} = 0.

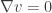Consequently, v\in W^{1,p}(\Omega) with \nabla v=0 a.e. Thus v is constant since \Omega is connected. Since v_\Omega=0 then v \equiv 0. This contradicts to \|v\|_{L^p(\Omega)}=1.

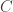The Poincaré inequality for W_0^{1,2}(\Omega) spaces. Assume that \Omega is a bounded open subset of the n-dimensional Euclidean space \mathbb R^n with a Lipschitz boundary (i.e., \Omega is an open, bounded Lipschitz domain). Then there exists a constant C, depending only on \Omega such that for every function u in the Sobolev space W_0^{1,2}(\Omega),

\displaystyle \| u \|_{L^2(\Omega)} \leq C \| \nabla u \|_{L^2(\Omega)}.

Proof. Assume \Omega can be enclosed in a cube

\displaystyle Q=\{ x \in \mathbb R^n: |x_i| \leqslant a, 1\leqslant i \leqslant n\}.

Then for any x \in Q, we have

\displaystyle\begin{gathered} {u^2}(x) = {\left( {\int_{ - a}^{{x_1}} {{u_{{x_1}}}(t,{x_2},...,{x_n})dt} } \right)^2} \hfill \\ \qquad\leqslant ({x_1} + a)\int_{ - a}^{{x_1}} {{{({u_{{x_1}}})}^2}dt} \hfill \\ \qquad\leqslant 2a\int_{ - a}^a {{{({u_{{x_1}}})}^2}dt} . \hfill \\ \end{gathered}.

Thus

\displaystyle\int_{ - a}^a {{u^2}(x)dx} \leqslant 4{a^2}\int_{ - a}^a {{{({u_{{x_1}}})}^2}dt}.

Integration over x_2,...,x_n from -a to a gives the result.

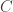The Poincaré inequality for W_0^{1,p}(\Omega) spaces. Assume that 1\leq p<n and that \Omega is a bounded open subset of the n-dimensional Euclidean space \mathbb R^n with a Lipschitz boundary (i.e., \Omega is an open, bounded Lipschitz domain). Then there exists a constant C, depending only on \Omega and p, such that for every function u in the Sobolev space W_0^{1,p}(\Omega),

\displaystyle \| u \|_{L^{p^\star} (\Omega)} \leq C \| \nabla u \|_{L^{p} (\Omega)},

where p^\star is defined to be \frac{np}{n-p}.

Proof. The proof of this version is exactly the same to the proof of W^{1,p}(\Omega) case.

Remark. The point u =0 on the boundary of \Omega is important. Otherwise, the constant function will not satisfy the Poincaré inequality. In order to avoid this restriction, a weight has been added like the classical Poincaré inequality for W^{1,p}(\Omega) case. Sometimes, the Poincaré inequality for W_0^{1,p}(\Omega) spaces is called the Sobolev inequality.

February 8, 2010

The two-dimensional isodiametric inequality

Filed under: Các Bài Tập Nhỏ, Giải Tích 2, Nghiên Cứu Khoa Học — Tags: — Ngô Quốc Anh @ 0:00

The isodiametric inequality states that, of all bodies of a given diameter, the sphere has the greatest volume. A proof can be found, e.g., in Lawrence C. Evans & Ronald F Gariepy: Measure theory and fine properties of functions. This note is about a particularly simple and beautiful proof of the isodiametric inequality in two dimensions.

I originally posted this as a plea for help to discover the origin of the proof. I have since learned that it is found in Littlewood’s miscellany, on p. 32.

The diameter of a body is defined as the supremum of the distances between two points in the body.

Clearly, we need only showthe isodiametric inequality for convex bodies, since taking the convex closure does not increase the diameter, nor does it decrease the area. Next, we may move the (convex) body so that it lies in the upper half plane, with the origin at its boundary. Thus we may describe the body in polar coordinates by

r\leqslant f(\theta), \quad 0\leqslant\theta\leqslant\pi.

We now apply a bit of first year calculus to write the area of the body as

\displaystyle A = \frac{1}{2}\int_0^\pi {f{{(\theta )}^2}d\theta }.

We now split the integral in two halves and change the variable in the second half so that both halves can be written as the single integral

\displaystyle A = \frac{1}{2}\int_0^{\frac{\pi }{2}} {\left( {f{{(\theta )}^2} + f{{\left( {\theta + \frac{\pi }{2}} \right)}^2}} \right)d\theta }.

But here we recognize the integrand as the squared hypothenuse of the right triangle in the figure. By definition, the hypothenuse cannot be greater than the diameter d of the region

\displaystyle f{(\theta )^2} + f{\left( {\theta + \frac{\pi }{2}} \right)^2} \leqslant {d^2}.

Thus

\displaystyle A \leqslant \pi {\left( {\frac{d}{2}} \right)^2}

which is the isodiametric inequality, and so the proof is complete.

Source: http://www.math.ntnu.no/~hanche/blog/isodiametric.pdf

January 17, 2010

New Inequalities of Ostrowski-like Type Involving n knots and L^p-norm of m-th derivative

Filed under: Nghiên Cứu Khoa Học — Tags: — Ngô Quốc Anh @ 22:58

Let me introduce my recent result with V.N. Huy published in Applied Mathematics Letters last year.

I want to start by recalling the following results due to Nenad Ujevic:

Let I \subset \mathbb R be an open interval such that [a,b] \subset I and let f : I \to \mathbb R be a twice differentiable function such that f'' is bounded and integrable. Then we have

\displaystyle\begin{gathered}\Bigg|\int\limits_a^b {f\left( x \right)dx}- \frac{{b - a}}{2}\Bigg(f\left( {\frac{{a + b}}{2} - \left( {2 - \sqrt 3 } \right)\left( {b - a} \right)} \right) \hfill \\ \qquad\qquad+ f\left( {\frac{{a + b}}{2} + \left( {2 - \sqrt 3 } \right)\left( {b - a} \right)} \right)\Bigg)\Bigg| \leqq \frac{{7 - 4\sqrt 3 }}{8}{\left\| {f''} \right\|_\infty }{\left( {b - a} \right)^3}. \hfill \\ \end{gathered}

And

Let I \subset \mathbb R be an open interval such that [a,b] \subset I and let f : I \to \mathbb R be a twice differentiable function such that f'' \in L^2(a,b). Then we have

\displaystyle\begin{gathered}\Bigg|\int\limits_a^b {f\left( x \right)dx}- \frac{{b - a}}{2}\Bigg(f\left( {\frac{{a + b}}{2} - \frac{{3 - \sqrt 6 }}{2}\left( {b - a} \right)} \right) \hfill \\ \qquad\qquad+ f\left( {\frac{{a + b}}{2} + \frac{{3 - \sqrt 6 }}{2}\left( {b - a} \right)} \right)\Bigg)\Bigg| \leqq \sqrt {\frac{{49}}{{80}} - \frac{1}{4}\sqrt 6 } {\left\| {f''} \right\|_2}{\left( {b - a} \right)^{\frac{5}{2}}}. \hfill \\ \end{gathered}

In the above mentioned results, constants \frac{{7 - 4\sqrt 3 }}{8} in the first and \sqrt {\frac{{49}}{{80}} - \frac{1}{4}\sqrt 6 } in the second result are sharp in sense that these cannot be replaced by smaller ones. This leads us to strengthen them by enlarging the number of knots (2 knots in both results) and replacing the norms \| \cdot \|_\infty in the first and \| \cdot \|_2 in the second.

Before stating our main result, let us introduce the following notation

\displaystyle I\left( f \right) =\int\limits _a^b {f\left( x \right)dx } .

Let 1 \leqq m, n<\infty and 1 \leqq p \leqq \infty. For each i = \overline{1,n}, we assume 0 < x_i < 1 such that

\displaystyle\left\{ \begin{gathered}{x_1} + {x_2} +\cdots+ {x_n} = \frac{n}{2}, \hfill \\ \cdots\hfill \\x_1^j + x_2^j +\cdots+ x_n^j = \frac{n}{{j + 1}}, \hfill \\ \cdots\hfill \\x_1^{m - 1} + x_2^{m - 1} +\cdots+ x_n^{m - 1} = \frac{n}{m}. \hfill \\ \end{gathered}\right.

Put

\displaystyle Q\left( {f,n,m,x_1 ,...,x_n } \right) = \frac{{b - a}}{n}\sum\limits_{i = 1}^n {f\left( {a + x_i \left( {b - a} \right)} \right)} .

We are in a position to state our main result.

Let I \subset \mathbb R be an open interval such that [a,b] \subset I and let f : I \to \mathbb R be a m-th differentiable function such that f^{(m)} \in L^p(a,b). Then we have

\displaystyle\left| {I\left( f \right) - Q\left( {f,n,m,{x_1},...,{x_n}} \right)} \right| \leqq \frac{1}{{m!}}\left( {{{\left( {\frac{1}{{mq + 1}}} \right)}^{\frac{1}{q}}} + {{\left( {\frac{1}{{\left( {m - 1} \right)q + 1}}} \right)}^{\frac{1}{q}}}} \right){\left\| {{f^{\left( m \right)}}} \right\|_p}{\left( {b - a} \right)^{m + \frac{1}{q}}}.

As can be seen the above result is not sharp. It will be very interesting if we can derive a sharp estimate. Note that, the results due to Nenad Ujevic can be rewritten as the following

\displaystyle\left| {I\left( f \right) - Q\left( {f,2,2,\frac{1}{2} - \left( {2 - \sqrt 3 } \right),\frac{1}{2} + \left( {2 - \sqrt 3 } \right)} \right)} \right| \leqq \frac{{7 - 4\sqrt 3 }}{8}{\left\| {f''} \right\|_\infty }{\left( {b - a} \right)^3}

and

\displaystyle\left| {I\left( f \right) - Q\left( {f,2,2,\frac{1}{2} - \frac{{3 - \sqrt 6 }}{2},\frac{1}{2} + \frac{{3 - \sqrt 6 }}{2}} \right)} \right| \leqq \sqrt {\frac{{49}}{{80}} - \frac{1}{4}\sqrt 6 } {\left\| {f''} \right\|_2}{\left( {b - a} \right)^{\frac{5}{2}}}.

August 29, 2009

On a polynomials of degree n, having all its zeros in the unit dis

Filed under: Các Bài Tập Nhỏ, Giải tích 7 (MA4247), Linh Tinh, Nghiên Cứu Khoa Học — Tags: — Ngô Quốc Anh @ 21:07

I found a very useful inequality involving a polynomials of degree n, having all its zeros in the unit disk from the following paper, doi:10.1016/j.jmaa.2009.07.049, published in J. Math. Anal. Appl.

Statement. If P(z) is a polynomial of degree n, having all its zeros in the disk |z| \leq 1, then

\left| {zP'(z)} \right| \geq \displaystyle\frac{n} {2}\left| {P(z)} \right|

for |z|=1.

Proof. Since all the zeros of P(z) lie in $latex|z| \leq 1$. Hence if z_1, z_2,...,z_n are the zeros of P(z), then |z_j| \leq 1 for all j =1,2,...,n. Clearly,

\displaystyle\Re \frac{{{e^{i\theta }}P'\left( {{e^{i\theta }}} \right)}}{{P\left( {{e^{i\theta }}} \right)}} = \sum\limits_{j = 1}^n {\Re \frac{{{e^{i\theta }}}}{{{e^{i\theta }} - {z_j}}}} ,

for every point e^{i\theta}, 0 \leq \theta < 2 \pi which is not a zero of P(z). Note that

\displaystyle\sum\limits_{j = 1}^n {\Re \frac{e^{i\theta }}{e^{i\theta } - z_j}}\geq\sum\limits_{j = 1}^n{\frac{1}{2}}=\frac{n}{2}.

This implies

\displaystyle\left| {\frac{{{e^{i\theta }}P'\left( {{e^{i\theta }}} \right)}}{{P\left( {{e^{i\theta }}} \right)}}} \right| \geq \Re \frac{{{e^{i\theta }}P'\left( {{e^{i\theta }}} \right)}}{{P\left( {{e^{i\theta }}} \right)}} \geq \frac{n}{2},

for every point e^{i \pi}, 0 \leq \theta < 2\pi. Hence

\left| {zP'(z)} \right| \geq \displaystyle \frac{n}{2}\left| {P(z)} \right|

for |z|=1 and this completes the proof.

Older Posts »

Blog at WordPress.com.