The inverse of the Laplace transform by contour integration

September 1, 2010

The inverse of the Laplace transform by contour integration

Filed under: Giải tích 7 (MA4247) — Ngô Quốc Anh @ 17:23

Usually, we can find the inverse of the Laplace transform $\mathcal L[\cdot](s)$ by looking it up in a table. In this entry, we show an alternative method that inverts Laplace transforms through the powerful method of contour integration.

Consider the piece-wise differentiable function $f(x)$ that vanishes for $x < 0$ . We can express the function $e^{-cx}f(x)$ by the complex Fourier representation of

$\displaystyle f(x){e^{ - cx}} = \frac{1}{{2\pi }}\int_{ - \infty }^\infty {{e^{i\omega x}}\left[ {\int_0^\infty {{e^{ - ct}}f(t){e^{ - i\omega t}}dt} } \right]d\omega }$

for any value of the real constant $c$ , where the integral

$\displaystyle I = \int_0^\infty {{e^{ - ct}}|f(t)|dt}$

exists. By multiplying both sides of first equation by $e^{cx}$ and bringing it inside the first integral

$\displaystyle f(x) = \frac{1}{{2\pi }}\int_{ - \infty }^\infty {{e^{(c + i\omega )x}}\left[ {\int_0^\infty {f(t){e^{ - (c + i\omega )t}}dt} } \right]d\omega }$ .

With the substitution $z = c+\omega i$ , where $z$ is a new, complex variable of integration,

$\displaystyle f(x) = \frac{1}{{2\pi }}\int_{c - \infty i}^{c + \infty i} {{e^{zx}}\left[ {\int_0^\infty {f(t){e^{ - zt}}dt} } \right]d\omega }$ .

The quantity inside the square brackets is the Laplace transform $\mathcal L[f](z)$ . Therefore, we can express $f(t)$ in terms of its transform by the complex contour integral

Theorem (Bromwich’s integral). The following line integral runs along the line $x = c$ parallel to the imaginary axis and $c$ units to the right of it, the so-called Bromwich contour

$\displaystyle f(t) = \frac{1}{{2\pi i}}\int_{c - \infty i}^{c + \infty i} {\mathcal L[f](z){e^{tz}}dz}$ .

Example. Let us invert

$\displaystyle L(s) = \frac{{{e^{ - 3s}}}}{{{s^2}(s - 1)}}$ .

Solution. From Bromwich’s integral,

$\displaystyle\begin{gathered} f(t) = \frac{1}{{2\pi i}}\int_{c - \infty i}^{c + \infty i} {\frac{{{e^{(t - 3)s}}}}{{{z^2}(z - 1)}}dz} \hfill \\ \qquad= \frac{1}{{2\pi i}}\oint_C {\frac{{{e^{(t - 3)s}}}}{{{z^2}(z - 1)}}dz} - \frac{1}{{2\pi i}}\int_{{C_R}} {\frac{{{e^{(t - 3)s}}}}{{{z^2}(z - 1)}}dz} \hfill \\ \end{gathered}$

where $C_R$ is a semicircle of infinite radius in either the right or left half of the $z$ -plane and $C$ is the closed contour that includes $C_R$ and Bromwich’s contour.

Our first task is to choose an appropriate contour so that the integral along $C_R$ vanishes. By Jordan’s lemma, this requires a semicircle in the right half-plane if $t-3 < 0$ and a semicircle in the left half-plane if $t- 3>0$ . Consequently, by considering these two separate cases, we force the second integral to zero and the inversion simply equals the closed contour.

Consider the case $t < 3$ first. Because Bromwich’s contour lies to the right of any singularities, there are no singularities within the closed contour and $f(t) = 0$ .

Consider now the case $t > 3$ . Within the closed contour in the left half-plane, there is a second-order pole at $z = 0$ and a simple pole at $z = 1$ . Therefore,

$\displaystyle f(t) = \mathop {\rm Res}\limits_{z = 0} \left[ {\frac{{{e^{(t - 3)s}}}}{{{z^2}(z - 1)}}} \right] + \mathop {\rm Res}\limits_{z = 1} \left[ {\frac{{{e^{(t - 3)s}}}}{{{z^2}(z - 1)}}} \right]$

where

$\displaystyle\mathop {\rm Res}\limits_{z = 0} \left[ {\frac{{{e^{(t - 3)s}}}}{{{z^2}(z - 1)}}} \right] = \mathop {\lim }\limits_{z \to 0} \frac{d}{{dz}}\left[ {{z^2}\frac{{{e^{(t - 3)s}}}}{{{z^2}(z - 1)}}} \right] = 2 - t$

and

$\displaystyle\mathop {\rm Res}\limits_{z = 1} \left[ {\frac{{{e^{(t - 3)s}}}}{{{z^2}(z - 1)}}} \right] = \mathop {\lim }\limits_{z \to 1} \frac{d}{{dz}}\left[ {(z - 1)\frac{{{e^{(t - 3)s}}}}{{{z^2}(z - 1)}}} \right] = {e^{t - 3}}$ .

Taking our earlier results into account, the inverse equals

$\displaystyle f(t) = \left[ {{e^{t - 3}} - (t - 3) - 1} \right]H(t - 3)$ .

Source: Green’s Functions with Applications by Dean G. Duffy

Comments (3)

3 Comments »

Excuse me, I have a question.
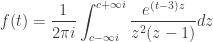
the first equality on the solution of the example, I think z have to be put instead of s.
like this
$\displaystyle f(t) = \frac{1}{2\pi i}\int_{c-\infty i}^{c+\infty i}\frac{e^{(t-3)z}}{z^2 (z-1)}dz$
Am I right?

Comment by zariski — September 10, 2010 @ 19:41

Reply
- Hi zariski.
  
  You are right, it also appears that $s$ in the second line need to be changed, i.e., the whole stuff reads as follows
  
  $\displaystyle\begin{gathered} f(t) = \frac{1}{{2\pi i}}\int_{c - \infty i}^{c + \infty i} {\frac{{{e^{(t - 3)z}}}}{{{z^2}(z - 1)}}dz} \hfill \\ \qquad= \frac{1}{{2\pi i}}\oint_C {\frac{{{e^{(t - 3)z}}}}{{{z^2}(z - 1)}}dz} - \frac{1}{{2\pi i}}\int_{{C_R}} {\frac{{{e^{(t - 3)z}}}}{{{z^2}(z - 1)}}dz}. \hfill \\ \end{gathered}$
  
  Thanks for visiting my blog and also pointing out the misprint.
  
  Comment by Ngô Quốc Anh — September 10, 2010 @ 21:27
  
  Reply
Thanks for this post. It was a useful overview.

Comment by Sunil — August 12, 2012 @ 9:10

Reply

RSS feed for comments on this post. TrackBack URI

M	T	W	T	F	S	S
		1	2	3	4	5
6	7	8	9	10	11	12
13	14	15	16	17	18	19
20	21	22	23	24	25	26
27	28	29	30

	Earth Salt and Space… on Stereographic projection
	Ngô Quốc Anh on Extending functions between me…
	Why does Paneitz ope… on Conformal Changes of Riemannia…
	Ngô Quốc Anh on Conformal Changes of Riemannia…
	NT Tài on Conformal Changes of Riemannia…
	Calculating scalar c… on Conformal change of the Laplac…
	Poincare inequality?… on The Poincaré inequality: W^{1,…
	Implicit function th… on The implicit function theorem:…
	Heuristics for the Y… on The Yamabe problem: A Sto…
	Is there a reference… on Compact embedding of Hölder…

Ngô Quốc Anh

September 1, 2010